
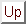

where
and
denote
the current value adjoint variables to
and
, respectively.
Note that we set
for further convenience.
As the optimal change
of the blood sucking rate
has to maximize the Hamiltonian, the assumptions made on the function
imply that
has to follow
and hence .
The time derivatives of the adjoint variables are given by
As the Hamiltonian (14) is concave jointly in the states and the control, the canonical system consisting of the two state equations (12) and (13), the adjoint equations (16) and (17) and the optimality condition (15) together with the limiting transversality condition
are not only necessary but even sufficient conditions for optimal solutions of our problem. Our aim is to show that the canonical system possesses stable periodic solutions. One way to do this is to apply the Hopf bifurcation theorem. Among other things this requires that the canonical system has an equilibrium and that the Jacobian evaluated at the steady state possesses a pair of purely imaginary eigenvalues and no other eigenvalue with zero real part.
First we want to establish the existence of a steady state. A steady
state
of the canonical system is a solution to the following system of nonlinear
equations:
From (20) and (23)
and
follow
immediately. Moreover, (19) and (22) yield the relations
From (24) and (21) it can be seen that the steady state value
has to be a solution of
Specifying
as a linear function
with
,
is monotonically increasing and therefore at most one steady state can
exist. Moreover, if
hold, one and only one equilibrium exists.